TWELFTH GÖKOVA
GEOMETRY / TOPOLOGY CONFERENCE
May 30 - June 04 (2005)
Gökova, Turkey
Invited speakers
| V.Batyrev | |
R.Bryant | |
I.Ciocan-Fontanine |
| S.Encinas | |
T.Etgu | |
S.Gukov |
| I.Hambleton | |
H.Hauser | |
K.Karu |
| H.King | |
M.Korkmaz | |
A.Kovalev |
| V.Manturov | |
K.Matsuki | |
G.Mikhalkin |
| L.Ng | |
Y.Ozan | |
B.Ozbagci |
| J.Park | |
M.Polyak | |
J.Rasmussen |
| S.Salur | |
O.Viro | |
J.Wlodarczyk |
Scientific Committee : G. Tian, R. Stern, C. Vafa, R. Kirby,
Y. Eliashberg, S. Akbulut
Organizing Commitee : T. Onder, T. Dereli, S. Kocak, S. Finashin
This conference is sponsored by International
Symposium Program of
TUBITAK (Turkish Scientific and
Technical Council) and NSF (National Science
Foundation)
.
The participants of 12th Gökova Geometry - Topology Conference
List of Talks
 |
| Herwig Hauser | |
Lecture I: Blowups (mini course)
Geometric and algebraic definition; coordinate expression; universal property; compatibility with localization, completion, base change, group actions, lifting of automorphisms, flags; exceptional components; composition of blowups; singular centers.
Lecture II: Transforms
Transforms of polynomials, functions, ideals, varieties; strict, weak, total, controlled transform; equivariance properties; persistence properties (regularity, normal crossings); commutation properties (sums, products, coefficients, smooth morphisms); behaviour of order, Hilbert-Samuel function, initial ideal under blowup.
Lecture III: Mobiles and their local setups
The concept of mobiles as an appropriate resolution datum; inductive construction of local setups of mobiles; transform of mobile under blowup; local resolution invariant defined through setups.
Lecture IV: Resolution of singularities
Resolution of varieties, ideals, mobiles; basic properties of strong resolutions; the proof in characteristic 0.
|
 |
| Kalle Karu | |
Lecture I: The cd-index of fans
It is well known that the number of cones of various dimensions in a complete simplicial fan can be encoded as the Betti numbers of the corresponding toric varitety. In an analogous way, the number of chains of cones in the fan can be encoded in a polynomial called the cd-index. Unlike the Betti numbers, the geometric meaning of the cd-index is much less understood. In this talk I will give a geometric interpretation of the cd-index, different from the usual combinatorial construction. Using this interpretation I will explain why the coefficients of the cd-index have to be non-negative, discuss some other similarities with Betti numbers, and if time permits, applications to conjectures of Charney, Davis and Gal.
Lecture II: Toric Residue mirror conjecture
The toric residue mirror conjecture of Batyrev and Materov
states that a rational function, the toric residue, can be expanded in a
Laurent series where the coefficients are integrals over certain moduli
spaces of curves. This conjecture can be viewed algebraically as a
relation between two rings -- the cohomology ring of a toric variety and
the homogeneous coordinate ring of another, mirror toric variety. In
this talk I will explain the algebra behind the conjecture.
|
 |
| Ionut Ciocan-Fontanine | |
A generalisation of the Hori-Vafa conjecture
A few years ago, Hori and Vafa conjectured that the
Landau-Ginzburg model mirror to the nonlinear sigma-model on a
Grassmannian can be obtained by "symmetrizing" the Landau-Ginzburg
model mirror to a product of projective spaces.In particular, this
conjecture predicts a precise relation between the J-functions
(generating functions for certain genus zero Gromov-Witten
invariants) of these varieties. This talk will describe joint work
with Aaron Bertram and Bumsig Kim in which we argue that the
appropriate general context for the above relationship is that of
twisted GW invariants of abelian and nonabelian GIT quotients. As a
concrete example, I will present a theorem giving closed formulas for
the J-functions of all isotropic partial flag varieties of classical
type.
|
 |
| Lenny Ng | |
Lecture 1: Contact geometry and submanifold invariants
We construct invariants of smooth embeddings and immersions usingcontact geometry. The approach involves conormal bundles andLegendrian contact homology. We give an application to plane curveswithout dangerous self-tangencies.
Lecture 2: Knot contact homology
We give a topological description of a knot invariant derived fromLegendrian contact homology. We discuss properties of the invariant,including relations with the Alexander polynomial and A-polynomial.
|
 |
| Jarek Wlodarczyk | |
Simple Hironaka resolution
Building upon work of Villamayor and Bierstone-Milman we give a proof of the canonical Hironaka principalization and desingularization in characteristic zero. We introduce here the idea of homogenized ideals which gives a priori the canonicity of algorithm and radically simplifies the proof.
|
 |
| Santiago Encinas | |
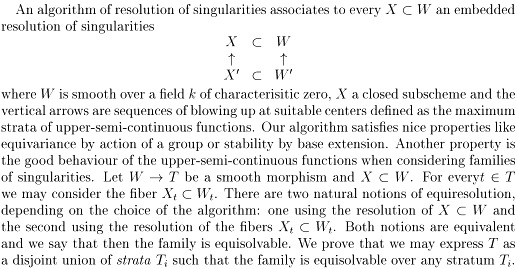
Algorithmic equiresolution
|
 |
| Robert Bryant | |
Lecture 1: Extension results for calibrations and special holonomy
Lecture 2: Calculations with G_2-structures
|
 |
| Jacob A Rasmussen | |
Khovanov Homology and Deformations
The Khovanov homology is a homological generalization of the Jones
polynomial. More recently, Khovanov and Rozanksy have defined a family of
similar theories generalizing the sl(N) polynomial of knots --- one for
each N>0. (The Jones polynomial corresponds to the case N=2.) Although the
definition of these theories is in principal combinatorial, they remain
rather poorly understood. I'll describe how the technique of deformation,
first introduced in the N=2 case by E.S. Lee, and studied in the sl(N)
case by Gornik, can be used to understand the structure of these theories
and to compute them for certain knots.
|
 |
| Victor Batyrev | |
Torsion in integral cohomology and mirror symmetry for Calabi-Yau 3-folds
We inverstigate the behavior of the torsion in the integral
cohomology of Calabi-Yau 3-folds under the mirror symmetry. There exist
473 800 776 families of Calabi-Yau 3-folds obtained from 4-dimensional
reflexive polytopes. For all these families we compute the integral
cohomology and show that the torsion in H2 and the torsion in H3
are exchanged by the mirror duality. We conjecture that this property
holds true for any mirror pair of Calabi-Yau 3-folds.
|
 |
| Sergei Gukov | |
The Geometry of Knot Homologies
|
 |
| Alexei Kovalev | |
Ricci-flat deformations of asymptotically cylindrical Calabi--Yau manifolds
We study a class of asymptotically cylindrical Ricci-flat Kähler
metrics arising on quasiprojective manifolds. Using the Calabi--Yau geometry
and analysis and building up on results of N.Koiso we show that under
rather general hypotheses `small' asymptotically cylindrical Ricci-flat
deformations of such metrics are again Kähler, with holonomy SU(n).
Further, the asymptotically cylindrical Ricci-flat Kähler metrics
corresponding to nearby complex structures and Kähler classes fill in
an open neighbourhood in the moduli space of asymptotically cylindrical
Ricci-flat metrics.
|
 |
| Oleg Viro | |
Alternatable virtual links
Virtual knots which can be made alternating by a change of some crossings are called alternatable. This property is not preserved by some second Reidemeister moves. Eliminating these moves gives rise a new kind of virtual links, intermediate between classical and virtual links.
Unlike virtual links, alternatable virtual links allow a natural extension of Khovanov homology with integer coefficients and signature.
|
 |
| Michael Polyak | |
Configuration spaces, homology intersections and finite type invariants
One may try to count (with certain multiplicities) various geometric objects inscribed into a generic curve immersed in Rn, so that their total number is preserved under homotopy in the class of generic immersions. The simplest examples include counting double tangents of a plane curve, or counting knot quadrisecants. In all cases invariants are easily seen to be of finite type. I will explain a general setting to produce such invariants (using certain evaluation maps of configuration spaces and homology intersections) and formulate some results and conjectures. If time permits, I will also discuss their relations to graph cohomology and Lie bialgebras.
|
 |
|

